|
|
|
|
|
|
|
  |
 Mar 21 2008, 03:29 PM Mar 21 2008, 03:29 PM
Publicado:
#1
|
|
 Dios Matemático Supremo Grupo: Usuario FMAT Mensajes: 1.920 Registrado: 19-August 06 Desde: DIM, DCC Beauchef Miembro Nº: 1.989 Nacionalidad:  Universidad:  Sexo:  |
Probar que
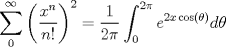 Mensaje modificado por neo shykerex el Mar 21 2008, 11:03 PM -------------------- Miembro de Anime No Seishin Doukokai, podrías ser el próximo.
|
|
|
|
 May 22 2008, 09:37 AM May 22 2008, 09:37 AM
Publicado:
#2
|
|
|
Doctor en Matemáticas Grupo: Usuario FMAT Mensajes: 156 Registrado: 11-June 07 Miembro Nº: 6.615 Nacionalidad:  Sexo:  |
![TEX: $$<br />\begin{gathered}<br /> {\text{Sea }}f\left( z \right) = \frac{{e^{x \cdot z} }}<br />{{z^{n + 1} }}{\text{ demostraremos primero que: res}}_{z = 0} f\left( z \right) = 2\pi \cdot i \cdot \left( {\tfrac{{x^n }}<br />{{n!}}} \right) \hfill \\<br /> {\text{En efecto}}{\text{, usando la expansi\'o n de la exponencial:}} \hfill \\<br /> f\left( z \right) = \frac{{1 + x \cdot z + \tfrac{{x^2 \cdot z^2 }}<br />{2} + ...}}<br />{{z^{n + 1} }} = z^{ - \left( {n + 1} \right)} + x \cdot z^{ - n} + ... + \underbrace {\left( {\tfrac{{x^n }}<br />{{n!}}} \right)}_{{\text{res}}_{z = 0} f\left( z \right)} \cdot z^{ - 1} + ... \hfill \\<br /> {\text{Por lo tanto para cualquier contorno }}\Gamma {\text{ que tenga al cero dentro de \'e l:}} \hfill \\<br /> \to \oint_\Gamma {f\left( z \right)dz} = 2\pi \cdot i \cdot \left( {\tfrac{{x^n }}<br />{{n!}}} \right) \hfill \\<br /> {\text{Ahora multiplicamos por }}\left( {\tfrac{{x^n }}<br />{{n!}}} \right){\text{ amos miembros: }}\oint_\Gamma {\left( {\tfrac{{x^n }}<br />{{n!}}} \right) \cdot \frac{{e^{x \cdot z} }}<br />{{z^{n + 1} }}dz} = 2\pi \cdot i \cdot \left( {\tfrac{{x^n }}<br />{{n!}}} \right)^2 \hfill \\<br /> {\text{Sumando: }}2\pi \cdot i \cdot \sum\limits_{n = 0}^\infty {\left( {\tfrac{{x^n }}<br />{{n!}}} \right)^2 } = \sum\limits_{n = 0}^\infty {\oint_\Gamma {\left( {\tfrac{{x^n }}<br />{{n!}}} \right) \cdot \frac{{e^{x \cdot z} }}<br />{{z^{n + 1} }}dz} } = \oint_\Gamma {\sum\limits_{n = 0}^\infty {\left( {\tfrac{{x^n }}<br />{{n!}}} \right) \cdot \frac{{e^{x \cdot z} }}<br />{{z^{n + 1} }}} dz} = \oint_\Gamma {\tfrac{{e^{{\raise0.5ex\hbox{$\scriptstyle x$}<br />\kern-0.1em/\kern-0.15em<br />\lower0.25ex\hbox{$\scriptstyle z$}}} \cdot e^{x \cdot z} }}<br />{z}dz} \hfill \\<br /> {\text{Ahora sea }}\Gamma {\text{ una circunferencia de radio 1 dada por: }}g\left( t \right) = e^{i \cdot t} ,0 \leqslant t \leqslant 2 \cdot \pi \hfill \\<br /> 2\pi \cdot i \cdot \sum\limits_{n = 0}^\infty {\left( {\tfrac{{x^n }}<br />{{n!}}} \right)^2 } = \oint_\Gamma {\tfrac{{e^{x \cdot z^{ - 1} } \cdot e^{x \cdot z} }}<br />{z}dz} = \int_0^\pi {\tfrac{{e^{x \cdot e^{ - it} } \cdot e^{x \cdot e^{it} } }}<br />{{e^{it} }}\left( {e^{it} } \right)^\prime dt} + \int_\pi ^{2\pi } {\tfrac{{e^{x \cdot e^{ - it} } \cdot e^{x \cdot e^{it} } }}<br />{{e^{it} }}\left( {e^{it} } \right)^\prime dt} \hfill \\<br /> 2\pi \cdot i \cdot \sum\limits_{n = 0}^\infty {\left( {\tfrac{{x^n }}<br />{{n!}}} \right)^2 } = i \cdot \left[ {\int_0^\pi {\tfrac{{e^{x \cdot e^{ - it} } \cdot e^{x \cdot e^{it} } }}<br />{{e^{it} }}e^{it} dt} + \int_\pi ^{2\pi } {\tfrac{{e^{x \cdot e^{ - it} } \cdot e^{x \cdot e^{it} } }}<br />{{e^{it} }}e^{it} dt} } \right] \hfill \\<br /> 2\pi \cdot \sum\limits_{n = 0}^\infty {\left( {\tfrac{{x^n }}<br />{{n!}}} \right)^2 } = \left[ {\int_0^\pi {e^{x \cdot \left( {e^{ - it} + e^{it} } \right)} dt} + \int_\pi ^{2\pi } {e^{x \cdot \left( {e^{ - it} + e^{it} } \right)} dt} } \right] \hfill \\<br /> {\text{Ahora recordemos que: }}e^{ - it} + e^{it} = 2 \cdot \cos \left( t \right) \hfill \\<br /> {\text{Entonces:}}{\text{ }}2\pi \cdot \sum\limits_{n = 0}^\infty {\left( {\tfrac{{x^n }}<br />{{n!}}} \right)^2 } = \int_0^{2\pi } {e^{2 \cdot x \cdot \cos \left( t \right)} dt} \hfill \\ <br />\end{gathered} <br />$$](./tex/19e891852c20be29bbd6c9e859ea9887.png) Saludos Mensaje modificado por PaulRS el May 22 2008, 09:49 AM -------------------- ![TEX: $\sqrt[3]{\displaystyle\sum_{i=1}^n{i^{3\cdot{\sqrt[]{3}}-1}}}\approx{\displaystyle\sum_{i=1}^n{i^{\sqrt[]{3}-1}}}$](./tex/1187710902.gif) |
|
|
|
 May 25 2008, 11:00 PM May 25 2008, 11:00 PM
Publicado:
#3
|
|
 Dios Matemático Supremo Grupo: Usuario FMAT Mensajes: 1.920 Registrado: 19-August 06 Desde: DIM, DCC Beauchef Miembro Nº: 1.989 Nacionalidad:  Universidad:  Sexo:  |
Una respuesta ejemplar, pasar a resueltos.
Saludos -------------------- Miembro de Anime No Seishin Doukokai, podrías ser el próximo.
|
|
|
|
 Jun 1 2008, 01:17 AM Jun 1 2008, 01:17 AM
Publicado:
#4
|
|
 Principiante Matemático Grupo: Usuario FMAT Mensajes: 8 Registrado: 1-June 08 Miembro Nº: 25.355 Sexo:  |
Este es un caso especial de
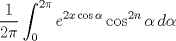 para cada para cada 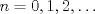 La demostración del caso general no necesita análisis complejo, existe un camino con herramientas reales. |
|
|
|
 Jun 1 2008, 10:41 AM Jun 1 2008, 10:41 AM
Publicado:
#5
|
|
|
Doctor en Matemáticas Grupo: Usuario FMAT Mensajes: 156 Registrado: 11-June 07 Miembro Nº: 6.615 Nacionalidad:  Sexo:  |
Este es un caso especial de 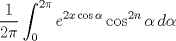 para cada para cada 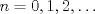 La demostración del caso general no necesita análisis complejo, existe un camino con herramientas reales. A ver... Sea 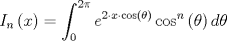 luego (Regla de Leibniz): luego (Regla de Leibniz): 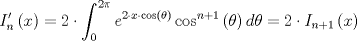 Ahora hallaremos la serie de Taylor de 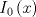 Según lo que acabamos de ver: 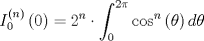 Además: 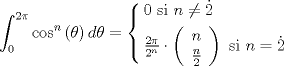 Luego: 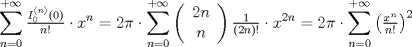 Así es que: 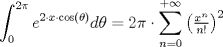 Saludos PD: Los demás casos se pueden hacer de la misma forma -------------------- ![TEX: $\sqrt[3]{\displaystyle\sum_{i=1}^n{i^{3\cdot{\sqrt[]{3}}-1}}}\approx{\displaystyle\sum_{i=1}^n{i^{\sqrt[]{3}-1}}}$](./tex/1187710902.gif) |
|
|
|
  |
1 usuario(s) está(n) leyendo esta discusión (1 invitado(s) y 0 usuario(s) anónimo(s))
0 miembro(s):
| Versión Lo-Fi | Fecha y Hora actual: 17th October 2025 - 05:51 AM |





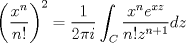 , donde
, donde 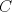 es cualquier curva encerrada en el origen
es cualquier curva encerrada en el origen 




