|
|
|
|
|
|
|
  |
 Jan 22 2006, 03:38 PM Jan 22 2006, 03:38 PM
Publicado:
#1
|
|
|
Webmaster Grupo: Administrador Mensajes: 6.692 Registrado: 13-May 05 Desde: Santiago Centro Miembro Nº: 2 Nacionalidad:  Sexo:  |
Sea
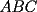 un triangulo acutangulo y un triangulo acutangulo y 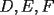 los pies de las alturas desde los pies de las alturas desde 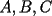 respectivamente. La recta que pasa por respectivamente. La recta que pasa por 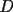 y es paralela a y es paralela a 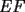 , corta a , corta a 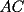 y a y a 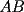 en en 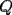 y y 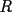 respectivamente.Sea respectivamente.Sea 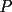 la interseccion de la interseccion de 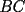 y y 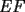 . Probar que la circunferencia circunscrita al triangulo . Probar que la circunferencia circunscrita al triangulo 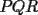 pasa a traves del punto medio de pasa a traves del punto medio de 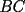 . .
-------------------- Colegios/Liceos/Universidades en Fmat (Integrate!!!!)
Videos PSU de Funciones (Y tú, ¿Aun estas aproblemado con Funciones?)   |
|
|
|
 Feb 27 2007, 05:34 PM Feb 27 2007, 05:34 PM
Publicado:
#2
|
|
 Dios Matemático Grupo: Usuario FMAT Mensajes: 374 Registrado: 16-September 06 Desde: New Haven, CT, USA. Miembro Nº: 2.275 Nacionalidad:  Colegio/Liceo:  Universidad:  Sexo:  |
Solución:
 screen.width*0.6) {this.resized=true; this.width=screen.width*0.4; this.alt='Pincha Aqui para ver esta imagen en su tamaño original';}" onmouseover="if(this.resized) this.style.cursor='hand';" onclick="if(this.resized) {window.open('http://img401.imageshack.us/img401/6672/lindomecostoxdcx3.png');}" /> screen.width*0.6) {this.resized=true; this.width=screen.width*0.4; this.alt='Pincha Aqui para ver esta imagen en su tamaño original';}" onmouseover="if(this.resized) this.style.cursor='hand';" onclick="if(this.resized) {window.open('http://img401.imageshack.us/img401/6672/lindomecostoxdcx3.png');}" />![TEX: \[<br />\begin{gathered}<br /> {\text{Sea M el punto medio de BC}}{\text{, unimos PQ}}{\text{, QM}}{\text{, MR}}{\text{, DE}}{\text{, DF}}{\text{,MF}}{\text{. Nombremos algunos angulos:}} \hfill \\<br /> \angle CAD{\text{ = }}\angle {\text{CBE = }}\angle EFH{\text{ = }}\angle HFD{\text{ = }}\alpha {\text{; }}\angle DAB{\text{ = }}\angle FCB{\text{ = }}\angle FEH{\text{ = }}\angle DEH{\text{ = }}\beta {\text{; }} \hfill \\<br /> \angle ACF{\text{ = }}\angle EBA{\text{ = }}\angle EDH{\text{ = }}\angle HDF{\text{ = }}\gamma {\text{ /}}\alpha + \beta + \gamma = 90{\text{ }} \hfill \\<br /> ({\text{todos estas relaciones vienen de los ciclicos}}). \hfill \\<br /> {\text{Notemos que }}\angle {\text{DEC = }}\angle {\text{AEF = }}\angle {\text{PEC = 90 - }}\beta \Rightarrow \angle AQR = {\text{90 - }}\beta \Leftrightarrow \angle {\text{EDR = 180 - 2}}\beta \hfill \\<br /> {\text{(paralelas)}}{\text{. Sea QR}} \cap {\text{EB = N}} \Rightarrow \angle {\text{ENQ = }}\beta \Rightarrow \boxed{DQ = DE = ND}(1) \hfill \\<br /> {\text{Tambien veamos que }}\angle {\text{DRF = }}\angle {\text{DFR = 90 - }}\alpha \Rightarrow \boxed{FD = RD}(2) \hfill \\<br /> {\text{Recordando la circunferencia de los 9 puntos sabemos que EDMF es ciclico}}{\text{.}} \hfill \\<br /> {\text{Como }}\angle {\text{EDC = 90 - }}\gamma {\text{ = }}\alpha + \beta = \angle EFM \Rightarrow \angle DFM = \beta - \alpha ,{\text{ pero como }}\angle PDE{\text{ = }}\angle FDM \hfill \\<br /> \Rightarrow \vartriangle PDE \sim \vartriangle FDM \Rightarrow \boxed{\frac{{PD}}<br />{{DE}} = \frac{{FD}}<br />{{DM}}}(3) \hfill \\<br /> {\text{Por (2) sabemos que }}\frac{{FD}}<br />{{{\text{DM}}}} = \frac{{RD}}<br />{{DM}} \Rightarrow \frac{{PD}}<br />{{DE}} = \frac{{RD}}<br />{{DM}},{\text{ por (1) : }}\boxed{\frac{{PD}}<br />{{DQ}} = \frac{{RD}}<br />{{DM}}} \hfill \\<br /> {\text{Luego como }}\angle PDQ{\text{ = }}\angle RDM \Rightarrow \vartriangle PDQ \sim \vartriangle RDM \Rightarrow \angle QPD{\text{ = }}\angle MRD \hfill \\<br /> {\text{Demostrando que PQMR es ciclico}}{\text{.}} \hfill \\ <br />\end{gathered} <br />\]<br />](./tex/1172615676.gif)
|
|
|
|
  |
1 usuario(s) está(n) leyendo esta discusión (1 invitado(s) y 0 usuario(s) anónimo(s))
0 miembro(s):
| Versión Lo-Fi | Fecha y Hora actual: 25th October 2025 - 01:26 PM |








