Destacada Desigualdad - Foro fmat.cl

Foro fmat.cl > Sector Preparación Olimpiadas > Competencias y Olimpiadas > Destacados FMAT > Las Mejores Soluciones

Destacada Desigualdad, Resuelta por Jaime SSCC

Opciones

Rurouni Kenshin Ver Perfil del Miembro Ver los mensajes de este miembro	Jan 19 2006, 11:16 PM Publicado: #1
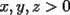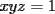Webmaster Grupo: Administrador Mensajes: 6.692 Registrado: 13-May 05 Desde: Santiago Centro Miembro Nº: 2 Nacionalidad: Sexo:	Problema Si $TEX: $x,y,z>0$$ con $TEX: $xyz=1$$ Probar que: 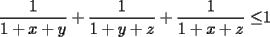$TEX: $\displaystyle \frac{1}{1+x+y}+\frac{1}{1+y+z}+\frac{1}{1+x+z}\le$$1$$ 18vo Torneo Internacional de las Ciudades Solucion: Tenemos que: $TEX: \noindent $(\sqrt[3]{x} - \sqrt[3]{y})^2 \ge 0$\\<br />$\sqrt[3]{x}^2 - 2\sqrt[3]{xy} + \sqrt[3]{y}^2 \ge 0$\\<br />$\sqrt[3]{x}^2 - \sqrt[3]{xy} + \sqrt[3]{y}^2 \ge \sqrt[3]{xy}$\\<br />\\<br />$\sqrt[3]{x}^3 + \sqrt[3]{y}^3 = (\sqrt[3]{x} + \sqrt[3]{y})(\sqrt[3]{x}^2 - \sqrt[3]{xy} + \sqrt[3]{y}^2) \ge \sqrt[3]{xy}(\sqrt[3]{x} + \sqrt[3]{y})$$ Asi Tenemos Que: $TEX: \noindent $\displaystyle \frac{1}{\sqrt[3]{x}^3 + \sqrt[3]{y}^3 + \sqrt[3]{xyz}} \le \frac{1}{\sqrt[3]{xy}(\sqrt[3]{x} + \sqrt[3]{y})+\sqrt[3]{xyz}} = \frac{1}{(\sqrt[3]{x} + \sqrt[3]{y} + \sqrt[3]{z})} \cdot \frac{1}{\sqrt[3]{xy}}$\\$ Remplazando $TEX: $\displaystyle \frac{1}{x + y + 1} \le \frac{1}{(\sqrt[3]{x} + \sqrt[3]{y} + \sqrt[3]{z})} \cdot \frac{1}{\sqrt[3]{xy}}$\\$ Analogamente $TEX: $\displaystyle \frac{1}{y + z + 1} \le \frac{1}{(\sqrt[3]{x} + \sqrt[3]{y} + \sqrt[3]{z})} \cdot \frac{1}{\sqrt[3]{yz}}$\\$ $TEX: $\displaystyle \frac{1}{z + x + 1} \le \frac{1}{(\sqrt[3]{x} + \sqrt[3]{y} + \sqrt[3]{z})} \cdot \frac{1}{\sqrt[3]{zx}}$\\$ Sumando $TEX: \noindent $\displaystyle \frac{1}{x + y + 1} + \frac{1}{y + z + 1} + \frac{1}{z + x + 1} \le \frac{1}{(\sqrt[3]{x} + \sqrt[3]{y} + \sqrt[3]{z})} \cdot (\frac{1}{\sqrt[3]{xy}} + \frac{1}{\sqrt[3]{yz}} + \frac{1}{\sqrt[3]{zx}})$\\$ $TEX: $\displaystyle \frac{1}{x + y + 1} + \frac{1}{y + z + 1} + \frac{1}{z + x + 1} \le \frac{1}{(\sqrt[3]{x} + \sqrt[3]{y} + \sqrt[3]{z})} \cdot \frac{(\sqrt[3]{x} + \sqrt[3]{y} + \sqrt[3]{z})}{\sqrt[3]{xyz}}$$ $TEX: $\displaystyle \frac{1}{x + y + 1} + \frac{1}{y + z + 1} + \frac{1}{z + x + 1} \le \frac{1}{\sqrt[3]{xyz}}$$ $TEX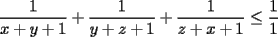: $\displaystyle \frac{1}{x + y + 1} + \frac{1}{y + z + 1} + \frac{1}{z + x + 1} \le \frac{1}{1}$$ Quedando demostrado Resuelto por Jaime SSCC -------------------- Colegios/Liceos/Universidades en Fmat (Integrate!!!!) Videos PSU de Funciones (Y tú, ¿Aun estas aproblemado con Funciones?)