Probando LaTeX - Foro fmat.cl

Portal Matemático

¿Qué es FMAT?

Foro fmat.cl > Sector Comunidad Fmat > Sector Manuales

437 Páginas:

« < 48 49 50 51 52 > »

Closed Topic

Start new topic

Probando LaTeX

karnack Ver Perfil del Miembro Ver los mensajes de este miembro	May 16 2007, 03:18 AM Publicado: #491
Dios Matemático Supremo Grupo: Usuario FMAT Mensajes: 691 Registrado: 2-December 06 Miembro Nº: 3.105	$TEX: $\mathit{hola} \mathbf{hola} \mathcal{abcdefghijklmnopqrstuvwxyz1234567890}$$

charly1234 Ver Perfil del Miembro Ver los mensajes de este miembro	May 16 2007, 06:00 PM Publicado: #492
Dios Matemático Grupo: Usuario FMAT Mensajes: 335 Registrado: 3-December 06 Desde: Viña del mar Miembro Nº: 3.111 Nacionalidad: Colegio/Liceo: Universidad: Sexo:	como se hace para hacer funciones esas que tienen el sigo {..} onda, f(x)={x+2;x>1,x-2;x<1} $TEX: \[<br />\begin{array}{l}<br /> f(x) = \left( {\begin{array}{{20}c}<br /> {x} & {;0 \le x \le 1} \\<br /> {[x]+1} & {;1 < x \le 10} \\<br />\end{array}} \right) \\ <br /> 30[\min ] = 1x \\ <br /> \end{array}<br />\]$ Mensaje modificado por charly1234* el May 16 2007, 06:20 PM --------------------

「Krizalid」 Ver Perfil del Miembro Ver los mensajes de este miembro	May 16 2007, 06:18 PM Publicado: #493
Staff FMAT Grupo: Super Moderador Mensajes: 8.124 Registrado: 21-May 06 Miembro Nº: 1.156 Nacionalidad: Sexo:	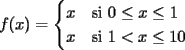$TEX: $f(x)=\begin{cases}<br />x&\text{si }0\le x\le1\\<br />x&\text{si }1<x\le10<br />\end{cases}$$ Código $f(x)=\begin{cases} x&\text{si }0\le x\le1\\ x&\text{si }1\<x\le10 \end{cases}$

charly1234 Ver Perfil del Miembro Ver los mensajes de este miembro	May 16 2007, 06:27 PM Publicado: #494
Dios Matemático Grupo: Usuario FMAT Mensajes: 335 Registrado: 3-December 06 Desde: Viña del mar Miembro Nº: 3.111 Nacionalidad: Colegio/Liceo: Universidad: Sexo:	aa, oka, vale --------------------

Fox Ver Perfil del Miembro Ver los mensajes de este miembro	May 17 2007, 10:03 PM Publicado: #495
Matemático Grupo: Usuario FMAT Mensajes: 39 Registrado: 10-January 07 Miembro Nº: 3.681 Nacionalidad: Universidad: Sexo:	$TEX: \[<br />\frac{{a \bullet \frac{{\sqrt {3 \bullet a} }}<br />{2}}}<br />{2}<br />\]$ Mensaje modificado por Fox el Jun 17 2007, 05:37 PM

Julio_fmat Ver Perfil del Miembro Ver los mensajes de este miembro	May 18 2007, 06:29 PM Publicado: #496
Dios Matemático Supremo Grupo: Usuario FMAT Mensajes: 4.874 Registrado: 19-January 07 Desde: Mathematics!! Miembro Nº: 3.830 Nacionalidad: Colegio/Liceo: Universidad: Sexo:	Y en este caso sucede lo mismo.... código: \[ \begin{gathered} \,\left( {\frac{1} {{36}}} \right)^{4 - 7x} \times 1296^{(2 + 5x)} = \,\left( {\frac{1} {{216}}} \right)^{2x + 7} :\,6^{1 - 9x} \hfill \\ 36^{ - 1(4 - 7x)} \times 6^4 ^{(2 + 5x)} = 216^{ - 1(2x + 7)} \times 6^{ - (1 - 9x)} \hfill \\ 36^{ - 4 + 7x} \times 6^{8 + 20x} = \,216^{ - 2x - 7} \times 6^{ - 1 + 9x} \hfill \\ 6^{2( - 4 + 7x)} \times 6^{8 + 20x} = \,6^{3( - 2x - 7)} \times 6^{ - 1 + 9x} \hfill \\ 6^{ - 8 + 14x} \times 6^{8 + 20x} = \,6^{ - 6x - 21} \times 6^{ - 1 + 9x} \hfill \\ 6^{ - 8 + 14x + 8 + 20x} = 6^{ - 6x - 21 + ( - 1 + 9x)} \hfill \\ 6^{34x} = 6^{ - 6x - 21 - 1 + 9x} \hfill \\ 6^{34x} = 6^{3x - 22} \hfill \\ 34x = 3x - 22 \hfill \\ 34x - 3x = - 22 \hfill \\ 31x = - 22 \hfill \\ x = \frac{{ - 22}} {{31}} \hfill \\ \end{gathered} \] -------------------- "... Lo veo, pero no puedo creerlo ... se trata de mostrar que las superficies, los volúmenes e incluso las variedades continuas de n dimensiones pueden ponerse en correspondencia unívoca con curvas continuas, o sea, con variedades de una sola dimensión, y que por consiguiente, las superficies, los volúmenes y las variedades de n dimensiones tienen también la misma potencia que las curvas ..." G. Cantor. Las Matemáticas son el lenguaje de la naturaleza, todo lo que nos rodea se puede representar y entender mediante números. Si se hace un gráfico con los números de un sistema, se forman modelos; éstos modelos están por todas partes en la naturaleza. Max Cohen. $TEX: $$\Phi=\displaystyle \int \limits_{-\infty}^x \dfrac{1}{\sqrt{2\pi}}e^{-\frac{1}{2}t^2}dt=\lim_{n\to +\infty}P\left(\dfrac{\displaystyle \sum_{i=1}^n x_i-n\mu}{n\sigma}\le x\right).$$$ Licenciado en Matemática (2021). Universidad de Concepción, Chile.

「Krizalid」 Ver Perfil del Miembro Ver los mensajes de este miembro	May 18 2007, 06:48 PM Publicado: #497
Staff FMAT Grupo: Super Moderador Mensajes: 8.124 Registrado: 21-May 06 Miembro Nº: 1.156 Nacionalidad: Sexo:	\[ \begin{gathered} \,\left( {\frac{1} {{36}}} \right)^{4 - 7x} \times 1296^{(2 + 5x)} = \,\left( {\frac{1} {{216}}} \right)^{2x + 7} :\,6^{1 - 9x} \hfill \\ 36^{ - 1(4 - 7x)} \times 6^4 ^{(2 + 5x)} = 216^{ - 1(2x + 7)} \times 6^{ - (1 - 9x)} \hfill \\ 36^{ - 4 + 7x} \times 6^{8 + 20x} = \,216^{ - 2x - 7} \times 6^{ - 1 + 9x} \hfill \\ 6^{2( - 4 + 7x)} \times 6^{8 + 20x} = \,6^{3( - 2x - 7)} \times 6^{ - 1 + 9x} \hfill \\ 6^{ - 8 + 14x} \times 6^{8 + 20x} = \,6^{ - 6x - 21} \times 6^{ - 1 + 9x} \hfill \\ 6^{ - 8 + 14x + 8 + 20x} = 6^{ - 6x - 21 + ( - 1 + 9x)} \hfill \\ 6^{34x} = 6^{ - 6x - 21 - 1 + 9x} \hfill \\ 6^{34x} = 6^{3x - 22} \hfill \\ 34x = 3x - 22 \hfill \\ 34x - 3x = - 22 \hfill \\ 31x = - 22 \hfill \\ x = \frac{{ - 22}} {{31}} \hfill \\ \end{gathered} \] Hay cosas demás. Por ejemplo \, eso es para generar espacio, pero no le veo la necesidad de emplearlo. Entiéndase que es copiar & pegar, solamente escribir lo que se debe escribir, no agregar cosas adicionales ya que puede ser esa la razón de que se generen los errores. $TEX: \[<br />\begin{gathered}<br /> \left( {\frac{1}<br />{{36}}} \right)^{4 - 7x} \times 1296^{(2 + 5x)} = \left( {\frac{1}<br />{{216}}} \right)^{2x + 7} \div 6^{1 - 9x} \hfill \\<br /> 36^{ - (4 - 7x)} \times 6^{4(2 + 5x)} = 216^{ - (2x + 7)} \times 6^{ - (1 - 9x)} \hfill \\<br /> 36^{7x - 4} \times 6^{8 + 20x} = 216^{ - 2x - 7} \times 6^{9x - 1} \hfill \\<br /> 6^{2(7x - 4)} \times 6^{8 + 20x} = 6^{3( - 2x - 7)} \times 6^{9x - 1} \hfill \\<br /> 6^{14x - 8} \times 6^{8 + 20x} = 6^{ - 6x - 21} \times 6^{9x - 1} \hfill \\<br /> 6^{34x} = 6^{3x - 22} \hfill \\<br /> 34x = 3x - 22 \hfill \\<br /> 31x = - 22 \hfill \\<br /> x = - \frac{{22}}<br />{{31}} \hfill \\ <br />\end{gathered} <br />\]$ \[ \begin{gathered} \left( {\frac{1} {{36}}} \right)^{4 - 7x} \times 1296^{(2 + 5x)} = \left( {\frac{1} {{216}}} \right)^{2x + 7} \div 6^{1 - 9x} \hfill \\ 36^{ - (4 - 7x)} \times 6^{4(2 + 5x)} = 216^{ - (2x + 7)} \times 6^{ - (1 - 9x)} \hfill \\ 36^{7x - 4} \times 6^{8 + 20x} = 216^{ - 2x - 7} \times 6^{9x - 1} \hfill \\ 6^{2(7x - 4)} \times 6^{8 + 20x} = 6^{3( - 2x - 7)} \times 6^{9x - 1} \hfill \\ 6^{14x - 8} \times 6^{8 + 20x} = 6^{ - 6x - 21} \times 6^{9x - 1} \hfill \\ 6^{34x} = 6^{3x - 22} \hfill \\ 34x = 3x - 22 \hfill \\ 31x = - 22 \hfill \\ x = - \frac{{22}} {{31}} \hfill \\ \end{gathered} \]

yolegendario Ver Perfil del Miembro Ver los mensajes de este miembro	May 19 2007, 01:02 AM Publicado: #498
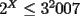
Principiante Matemático Grupo: Usuario FMAT Mensajes: 7 Registrado: 21-February 07 Miembro Nº: 4.143 Nacionalidad: Sexo:	$TEX: VIVALATEX$ $TEX: $2^X\le 3^2007$$

renito Ver Perfil del Miembro Ver los mensajes de este miembro	May 19 2007, 03:16 PM Publicado: #499
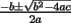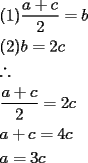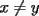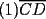
Dios Matemático Grupo: Usuario FMAT Mensajes: 341 Registrado: 9-February 07 Desde: valparaiso, chile Miembro Nº: 4.019 Nacionalidad: Universidad: Sexo:	$TEX: $\frac{{-b\pm\sqrt{b^2-4ac}}}{{2a}}$$ $TEX: $\begin{gathered}(1)\frac{{a+c}}{2}=b\hfill\\(2)b=2c\hfill\\\therefore\hfill\\\frac{{a+c}}{2}=2c\hfill\\a+c=4c\hfill\\a=3c\hfill\\\end{gathered}$$ $TEX: $x\not=y$$ $TEX: $(1)\overline{CD}$$ Mensaje modificado por renito el May 19 2007, 05:44 PM

renito Ver Perfil del Miembro Ver los mensajes de este miembro	May 19 2007, 03:22 PM Publicado: #500
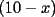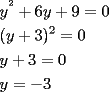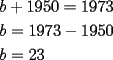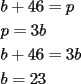
Dios Matemático Grupo: Usuario FMAT Mensajes: 341 Registrado: 9-February 07 Desde: valparaiso, chile Miembro Nº: 4.019 Nacionalidad: Universidad: Sexo:	$TEX: $(10-x)$$ $TEX: $\begin{gathered}y^{^2}+6y+9=0\hfill\\(y+3)^2=0\hfill\\y+3=0\hfill\\y=-3\hfill\\\end{gathered}$$ $TEX: $\begin{gathered}b+1950=1973\hfill\\b=1973-1950\hfill\\b=23\hfill\\\end{gathered}$$ $TEX: $\begin{gathered}b+46=p\hfill\\p=3b\hfill\\b+46=3b\hfill\\b=23\hfill\\\end{gathered}$$ Mensaje modificado por renito el May 19 2007, 07:02 PM

« Posterior · Sector Manuales · Anterior »

437 Páginas:

« < 48 49 50 51 52 > »

Closed Topic

Start new topic

1 usuario(s) está(n) leyendo esta discusión (1 invitado(s) y 0 usuario(s) anónimo(s))

0 miembro(s):

Modo de Ver: Estándar · Cambiar a: Lineal+ · Cambiar a: Outline

Suscribirse · Enviar por correo · Imprimir · Suscribirse a este foro

Versión Lo-Fi

Fecha y Hora actual: 26th November 2024 - 12:29 AM

Powered By IP.Board 2.2.1 © 2007 IPS, Inc.