Probando LaTeX - Foro fmat.cl

Portal Matemático

¿Qué es FMAT?

Foro fmat.cl > Sector Comunidad Fmat > Sector Manuales

437 Páginas:

« < 305 306 307 308 309 > »

Closed Topic

Start new topic

Probando LaTeX

mikelvader Ver Perfil del Miembro Ver los mensajes de este miembro	Jul 10 2009, 09:42 PM Publicado: #3061
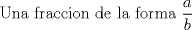
Maestro Matemático Grupo: Usuario FMAT Mensajes: 79 Registrado: 10-January 07 Miembro Nº: 3.694 Nacionalidad: Colegio/Liceo: Universidad: Sexo:	$TEX: Una fraccion de la forma $\displaystyle \frac{a}{b}$$ -------------------- Estudiante Ingenieria Civil Eléctrica EL QUE NADA SABE NADA TEME

ajenjo	Jul 12 2009, 04:43 PM Publicado: #3062
Invitado	$TEX: \[<br />\begin{gathered}<br /> \left. {\underline {\, <br /> \begin{gathered}<br /> \left( {x - y} \right)\left( {x^2 - y^2 } \right) = 160 \hfill \\<br /> \left( {x + y} \right)\left( {x^2 + y^2 } \right) = 580 \hfill \\ <br />\end{gathered} \,}}\! \right\| \hfill \\<br /> \hfill \\<br /> 1. - \hfill \\<br /> (x - y)(x + y)(x - y) = 160 \hfill \\<br /> (x + y)(x^2 - 2xy + y^2 ) = 160 \hfill \\<br /> x^3 - 2x^2 y + xy^2 + x^2 y - 2xy^2 + y^3 = 160 \hfill \\<br /> x^3 - x^2 y - xy^2 + y^3 = 160 \hfill \\<br /> \hfill \\<br /> 2. - \hfill \\<br /> (x + y)(x^2 + y^2 ) = 580 \hfill \\<br /> x^3 + xy^2 + x^2 y + y^3 = 580 \hfill \\<br /> \hfill \\<br /> Luego: \hfill \\<br /> \left. {\underline {\, <br /> \begin{gathered}<br /> x^3 - x^2 y - xy^2 + y^3 - 160 = 0 \hfill \\<br /> x^3 + xy^2 + x^2 y + y^3 - 580 = 0 \hfill \\ <br />\end{gathered} \,}}\! \right\| \hfill \\<br /> \hfill \\<br /> - 2(x^2 y + xy^2 - 210) = 0 \hfill \\<br /> x^2 y + xy^2 = 210 \hfill \\<br /> xy(x + y) = 210 \hfill \\<br /> xy(x + y) = 21 \bullet 10 \hfill \\<br /> \hfill \\<br /> \left. {\underline {\, <br /> \begin{gathered}<br /> xy = 10 \hfill \\<br /> x + y = 21 \hfill \\ <br />\end{gathered} \,}}\! \right\| \Rightarrow {\text{no hay solucion entera}} \hfill \\<br /> \left. {\underline {\, <br /> \begin{gathered}<br /> xy = 21 \hfill \\<br /> x + y = 10 \hfill \\ <br />\end{gathered} \,}}\! \right\| \Rightarrow 7,3 \hfill \\<br /> \hfill \\<br /> \boxed\begin{gathered}<br /> x = 7 \hfill \\<br /> y = 3 \hfill \\ <br />\end{gathered} \hfill \\ <br />\end{gathered} <br />\]$ Mensaje modificado por ajenjo el Jul 24 2009, 06:44 PM

xupurih Ver Perfil del Miembro Ver los mensajes de este miembro	Jul 12 2009, 10:24 PM Publicado: #3063
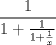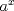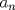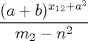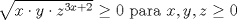Principiante Matemático Grupo: Usuario FMAT Mensajes: 7 Registrado: 22-October 08 Miembro Nº: 36.772 Nacionalidad: Universidad: Sexo:	$TEX: Prueba de Latex$ $TEX: $\displaystyle \frac {5}{3}$$ $TEX: $\displaystyle \frac {1}{1+\frac {1}{1+\frac{1}{x}}} $$ $TEX: $a^{x}$$ $TEX: $a_{n}$$ $TEX: $\displaystyle \frac {(a+b)^{x_{12}+a^{2}}}{m_{2}-n^{2}}$$ $TEX: $\sqrt{x \cdot y \cdot z^{3x+2}}$ $\ge 0$ para $x,y,z \ge 0$$ $TEX: $\sqrt[6]{x^{6}}$ = x$ $TEX: $\sqrt{1+\sqrt[3]{2+\sqrt[4]{3+\sqrt[5]{4}}}}$$ $TEX: $\overline {Yo \ se \ usar \ Latex}$ $\Rightarrow$ No Se Que Estoy Haciendo$ $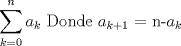TEX: $\displaystyle \sum_{k=0}^n a_{k} $ Donde $a_{k+1}$ = n-$a_{k}$$ $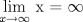TEX: $\displaystyle \lim_{x\rightarrow \infty}$ x = $\infty$$ $TEX: Fin Prueba de Latex (Aprendi <img src="style_emoticons/default/biggrin.gif" style="vertical-align:middle" emoid=":D" border="0" alt="biggrin.gif" />!)$

Kamufler Ver Perfil del Miembro Ver los mensajes de este miembro	Jul 14 2009, 12:41 PM Publicado: #3064
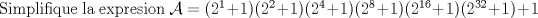
Dios Matemático Grupo: Usuario FMAT Mensajes: 277 Registrado: 15-October 08 Desde: Santiagopolis Miembro Nº: 36.209 Nacionalidad: Colegio/Liceo: Universidad: Sexo:	$TEX: \noindent Simplifique la expresion $\mathcal{A}=(2^1+1)(2^2+1)(2^4+1)(2^8+1)(2^{16}+1)(2^{32}+1)+1$$ --------------------

Kura Ver Perfil del Miembro Ver los mensajes de este miembro	Jul 15 2009, 06:55 PM Publicado: #3065
Dios Matemático Supremo Grupo: Usuario FMAT Mensajes: 1.918 Registrado: 14-May 08 Desde: The Tower of God Miembro Nº: 23.100 Nacionalidad: Colegio/Liceo: Universidad: Sexo:	$TEX: \noindent Se entiende como sustitución de Weierstrass lo siguiente:<br />\[u=\tan{\left(\dfrac{x}{2}\right)} \Rightarrow du= \dfrac{1}{2}\sec^2{\left(\frac{x}{2}\right)}dx\]<br />\noindent Luego para $-\pi < x < \pi$, y usando que:<br /> \[\tan{\left(\dfrac{x}{2}\right)}=\dfrac{\sin{\left(\dfrac{x}{2}\right)}}{\cos{\left(\dfrac{x}{2}\right)}} \]<br />\noindent Sabiendo que $\sin{\left(\dfrac{x}{2}\right)} = \sqrt{\dfrac{1-\cos{x}}{2}}$ y que $\cos{\left(\dfrac{x}{2}\right)}= \sqrt{\dfrac{1+\cos{x}}{2}}$ se obtiene:<br />\[\tan{\left(\dfrac{x}{2}\right)} = u=\dfrac { \sqrt{\dfrac{1-\cos{x}}{2}}} {\sqrt{\dfrac{1+\cos{x}}{2}}}\]<br />\noindent Luego: \[ u^2=\dfrac{1-\cos{x}}{1+\cos{x}} \Rightarrow \cos{x} = \dfrac{1-u^2}{1+u^2} \]<br />\noindent Usando Pitágoras obtenemos:<br />\[ \sin{x}= \dfrac{2u}{1+u^2}\]<br />\noindent Luego como $\sec{x}=\dfrac{1}{\cos{x}}$ y como $\cos{\left(\dfrac{x}{2}\right)} = \sqrt{\dfrac{1+\cos{x}}{2}}$ se tiene que: \[dx=\dfrac{2du}{1+u^2}\]<br />Esto finalmente nos permite transformar funciones racionales trigonométricas en funciones racionales ordinarias.<br />$ Ejemplo en acción: 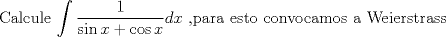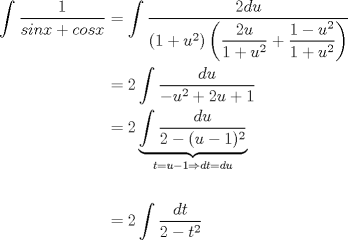$TEX: Calcule $\displaystyle\int \dfrac{1}{\sin{x}+\cos{x}}dx$ ,para esto convocamos a Weierstrass$ $TEX: \begin{equation}\begin{aligned}<br />\displaystyle\int \dfrac{1}{sin{x}+cos{x}}&= \int \dfrac{2du}{(1+u^2)\left(\dfrac{2u}{1+u^2} + \dfrac{1-u^2}{1+u^2}\right)} \\<br />&= 2\int \dfrac{du}{-u^2+2u+1} \\<br />&= 2\underbrace{\int \dfrac{du}{2-(u-1)^2}}_{t=u-1 \Rightarrow dt=du} \\<br />\\<br />&= 2 \int \dfrac{dt}{2-t^2}<br />\end{aligned}\end{equation}$ Esta ultima se resuelve, usando Sustitución Trigonométrica. Pero eso para la otra. - Mensaje modificado por Kura el Jul 15 2009, 07:12 PM -------------------- Far over... the misty mountains cold. To dungeons deep and caverns old. We must away ere break of day. To find our long-forgotten gold. The pines were roaring on the height. The winds were moaning in the night. The fire was red, it flaming spread. The trees like torches blazed with light. Apunte: Sistemas de Ecuaciones Cuadráticas! Apunte: Series de Fourier! Problemas Resueltos: EDO! OMG! Soy el ñoño de eléctrica.

daniel Ver Perfil del Miembro Ver los mensajes de este miembro	Jul 15 2009, 10:19 PM Publicado: #3066
Maestro Matemático Grupo: Usuario FMAT Mensajes: 70 Registrado: 19-April 06 Desde: Concepción - Chile Miembro Nº: 882 Nacionalidad: Sexo:	$TEX: $A = \frac{{\left[ {f(0) + f({x_1})} \right]\left( {{x_1} - 0} \right)}}{2}$$

jocast Ver Perfil del Miembro Ver los mensajes de este miembro	Jul 16 2009, 01:33 AM Publicado: #3067
Principiante Matemático Grupo: Usuario FMAT Mensajes: 1 Registrado: 6-July 09 Miembro Nº: 55.243	$TEX: $\sum_{k=1}^{n}x_{k}y_{k}=\left( \sum_{k=1}^{n}x_{k}^{2}\right) ^{\frac{1}{2}}\left( \sum_{k=1}^{n}y_{k}^{2}\right) ^{\frac{1}{2}}$$

LorDK Ver Perfil del Miembro Ver los mensajes de este miembro	Jul 16 2009, 04:01 PM Publicado: #3068
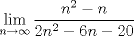
Dios Matemático Grupo: Usuario FMAT Mensajes: 311 Registrado: 18-June 08 Desde: Maipú Miembro Nº: 27.609 Nacionalidad: Colegio/Liceo: Universidad: Sexo:	$TEX: $ \displaystyle \lim_{n\rightarrow \infty} \dfrac {n^2 - n}{2n^2 - 6n - 20} $$ Mensaje modificado por LorDK el Jul 16 2009, 04:14 PM -------------------- Estudiante 4º año Licenciatura en Química Universidad de Santiago de Chile - USACH Astronomy Fan - LorDK

LorDK Ver Perfil del Miembro Ver los mensajes de este miembro	Jul 16 2009, 04:05 PM Publicado: #3069
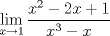
Dios Matemático Grupo: Usuario FMAT Mensajes: 311 Registrado: 18-June 08 Desde: Maipú Miembro Nº: 27.609 Nacionalidad: Colegio/Liceo: Universidad: Sexo:	$TEX: $\displaystyle \lim_{x\rightarrow 1} \dfrac {x^2 - 2x + 1}{x^3 - x}$$ Mensaje modificado por LorDK el Jul 16 2009, 04:05 PM -------------------- Estudiante 4º año Licenciatura en Química Universidad de Santiago de Chile - USACH Astronomy Fan - LorDK

LorDK Ver Perfil del Miembro Ver los mensajes de este miembro	Jul 16 2009, 04:11 PM Publicado: #3070
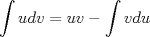
Dios Matemático Grupo: Usuario FMAT Mensajes: 311 Registrado: 18-June 08 Desde: Maipú Miembro Nº: 27.609 Nacionalidad: Colegio/Liceo: Universidad: Sexo:	$TEX: $ \displaystyle \int udv = uv - \displaystyle \int vdu$$ -------------------- Estudiante 4º año Licenciatura en Química Universidad de Santiago de Chile - USACH Astronomy Fan - LorDK

« Posterior · Sector Manuales · Anterior »

437 Páginas:

« < 305 306 307 308 309 > »

Closed Topic

Start new topic

5 usuario(s) está(n) leyendo esta discusión (5 invitado(s) y 0 usuario(s) anónimo(s))

0 miembro(s):

Modo de Ver: Estándar · Cambiar a: Lineal+ · Cambiar a: Outline

Suscribirse · Enviar por correo · Imprimir · Suscribirse a este foro

Versión Lo-Fi

Fecha y Hora actual: 18th February 2025 - 04:56 PM

Powered By IP.Board 2.2.1 © 2007 IPS, Inc.