Probando LaTeX - Foro fmat.cl

437 Páginas:

« < 258 259 260 261 262 > »

Probando LaTeX

Opciones

Mordurx Ver Perfil del Miembro Ver los mensajes de este miembro	Sep 5 2008, 05:57 PM Publicado: #2591
Principiante Matemático Grupo: Usuario FMAT Mensajes: 4 Registrado: 26-August 06 Desde: temuco Miembro Nº: 2.057 Nacionalidad: Universidad: Sexo:	[tex]noindent test [/text] Mensaje modificado por Mordurx el Sep 5 2008, 06:06 PM

Mordurx Ver Perfil del Miembro Ver los mensajes de este miembro	Sep 5 2008, 06:03 PM Publicado: #2592
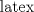
Principiante Matemático Grupo: Usuario FMAT Mensajes: 4 Registrado: 26-August 06 Desde: temuco Miembro Nº: 2.057 Nacionalidad: Universidad: Sexo:	$TEX: latex$ Mensaje modificado por Mordurx el Sep 5 2008, 06:06 PM

BENJAPISTOLAS Ver Perfil del Miembro Ver los mensajes de este miembro	Sep 6 2008, 01:50 AM Publicado: #2593
Dios Matemático Grupo: Usuario FMAT Mensajes: 283 Registrado: 5-September 08 Desde: Las Vegas Miembro Nº: 34.005 Nacionalidad: Colegio/Liceo: Sexo:	$TEX: $A\cup B$$

Todo sea por la ... Todo sea por la causa Ver Perfil del Miembro Ver los mensajes de este miembro	Sep 6 2008, 02:00 PM Publicado: #2594
Dios Matemático Grupo: Usuario FMAT Mensajes: 270 Registrado: 7-August 07 Miembro Nº: 8.312 Nacionalidad: Colegio/Liceo: Universidad: Sexo:	P10 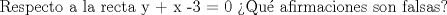$TEX: Respecto a la recta y + x -3 = 0 ¿Qué afirmaciones son falsas?$ Despejando Y = -x + 3 I) La recta intersecta al eje y en el punto (0,-3) Falso pues y= -0 +3 = 3 II) La recta tiene pendiente negativa Verdadero, de la ecuación despejada se desprende que la pendiente es -1 III) La recta pasa por el punto (2,1) Verdadero 2 = -1 + 3 Alternativa A Mensaje modificado por Todo sea por la causa el Sep 6 2008, 03:59 PM -------------------- Llegó el momento de la ansiada despedida :=)

topo Ver Perfil del Miembro Ver los mensajes de este miembro	Sep 6 2008, 03:39 PM Publicado: #2595
Dios Matemático Grupo: Usuario FMAT Mensajes: 380 Registrado: 17-March 08 Miembro Nº: 17.131 Nacionalidad: Colegio/Liceo: Universidad: Sexo:	$TEX: $\<br />\begin{gathered}<br /> \boxed{problema\,\,2} \hfill \\<br /> f(x + 1) = (x + 1)^2 ^{} + 3(x + 1) - 4 \hfill \\<br /> f(x - 1) = x^2 + 2x + 1 + 3x + 3 - 4 \hfill \\<br /> f(x - 1) = x^2 + 5x \hfill \\<br /> \boxed{alternativa\,\,A} \hfill \\ <br />\end{gathered} <br />\<br />$$

topo Ver Perfil del Miembro Ver los mensajes de este miembro	Sep 6 2008, 03:39 PM Publicado: #2596
Dios Matemático Grupo: Usuario FMAT Mensajes: 380 Registrado: 17-March 08 Miembro Nº: 17.131 Nacionalidad: Colegio/Liceo: Universidad: Sexo:	$TEX: $<br />\begin{gathered}<br /> \boxed{problema\,\,2} \hfill \\<br /> f(x + 1) = (x + 1)^2 ^{} + 3(x + 1) - 4 \hfill \\<br /> f(x - 1) = x^2 + 2x + 1 + 3x + 3 - 4 \hfill \\<br /> f(x - 1) = x^2 + 5x \hfill \\<br /> \boxed{alternativa\,\,A} \hfill \\ <br />\end{gathered} <br />\<br />$$

topo Ver Perfil del Miembro Ver los mensajes de este miembro	Sep 6 2008, 03:40 PM Publicado: #2597
Dios Matemático Grupo: Usuario FMAT Mensajes: 380 Registrado: 17-March 08 Miembro Nº: 17.131 Nacionalidad: Colegio/Liceo: Universidad: Sexo:	$TEX: $\[<br />\begin{gathered}<br /> \boxed{problema\,\,2} \hfill \\<br /> f(x + 1) = (x + 1)^2 ^{} + 3(x + 1) - 4 \hfill \\<br /> f(x - 1) = x^2 + 2x + 1 + 3x + 3 - 4 \hfill \\<br /> f(x - 1) = x^2 + 5x \hfill \\<br /> \boxed{alternativa\,\,A} \hfill \\ <br />\end{gathered} <br />\]<br />$$

topo Ver Perfil del Miembro Ver los mensajes de este miembro	Sep 6 2008, 03:40 PM Publicado: #2598
Dios Matemático Grupo: Usuario FMAT Mensajes: 380 Registrado: 17-March 08 Miembro Nº: 17.131 Nacionalidad: Colegio/Liceo: Universidad: Sexo:	$TEX: $\<br />\begin{gathered}<br /> \boxed{problema\,\,2} \hfill \\<br /> f(x + 1) = (x + 1)^2 ^{} + 3(x + 1) - 4 \hfill \\<br /> f(x - 1) = x^2 + 2x + 1 + 3x + 3 - 4 \hfill \\<br /> f(x - 1) = x^2 + 5x \hfill \\<br /> \boxed{alternativa\,\,A} \hfill \\ <br />\end{gathered} <br />\<br />$$

topo Ver Perfil del Miembro Ver los mensajes de este miembro	Sep 6 2008, 03:43 PM Publicado: #2599
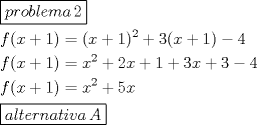
Dios Matemático Grupo: Usuario FMAT Mensajes: 380 Registrado: 17-March 08 Miembro Nº: 17.131 Nacionalidad: Colegio/Liceo: Universidad: Sexo:	$TEX: $<br />\begin{gathered}<br /> \boxed{problema\,2} \hfill \\<br /> f(x + 1) = (x + 1)^2 + 3(x + 1) - 4 \hfill \\<br /> f(x + 1) = x^2 + 2x + 1 + 3x + 3 - 4 \hfill \\<br /> f(x + 1) = x^2 + 5x \hfill \\<br /> \boxed{alternativa\,A} \hfill \\ <br />\end{gathered} <br />\<br />$$

Todo sea por la ... Todo sea por la causa Ver Perfil del Miembro Ver los mensajes de este miembro	Sep 6 2008, 04:08 PM Publicado: #2600
Dios Matemático Grupo: Usuario FMAT Mensajes: 270 Registrado: 7-August 07 Miembro Nº: 8.312 Nacionalidad: Colegio/Liceo: Universidad: Sexo:	P12 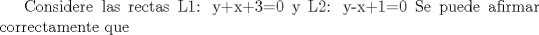$TEX: Considere las rectas L1: y+x+3=0 y L2: y-x+1=0 Se puede afirmar correctamente que$ L1:-x-3 L2: x -1 -------------------- Llegó el momento de la ansiada despedida :=)

437 Páginas:

« < 258 259 260 261 262 > »

1 usuario(s) está(n) leyendo esta discusión (1 invitado(s) y 0 usuario(s) anónimo(s))

0 miembro(s):

Modo de Ver: Estándar · Cambiar a: Lineal+ · Cambiar a: Outline

Fecha y Hora actual: 12th April 2025 - 07:24 AM