Probando LaTeX - Foro fmat.cl

Foro fmat.cl > Sector Comunidad Fmat > Sector Manuales

437 Páginas:

« < 253 254 255 256 257 > »

Probando LaTeX

Opciones

Andres villegas Ver Perfil del Miembro Ver los mensajes de este miembro	Aug 23 2008, 03:25 PM Publicado: #2541
Maestro Matemático Grupo: Usuario FMAT Mensajes: 115 Registrado: 20-May 08 Miembro Nº: 23.908 Nacionalidad: Colegio/Liceo: Universidad: Sexo:	$TEX: $\begin{gathered}<br /> P.7 \hfill \\<br /> \hfill \\<br /> f(x) = Ax^2 + Bx + C\xrightarrow{{}}{\text{ Funcion Cuadratica}}{\text{, C es donde la parabola corta al eje Y}} \hfill \\<br /> reemplazamos: \hfill \\<br /> \hfill \\<br /> Y = Px^2 - 3x + Q{\text{ intersecta al eje Y en el punto (0}}{\text{, - 4) e intersecta al eje X en el punto (4}}{\text{,0)}} \hfill \\<br /> \hfill \\<br /> 0 = 16P - 12 - 4 \hfill \\<br /> 16 = 16P \hfill \\<br /> 1 = P \hfill \\<br /> \hfill \\<br /> Entonces{\text{ P = 1 Q = - 4}} \hfill \\<br /> \hfill \\<br /> Alternativa{\text{ C}} \hfill \\ <br />\end{gathered} $<br />$ -------------------- Estudiante de Segundo Año Universidad Técnica Federico Santa María

Andres villegas Ver Perfil del Miembro Ver los mensajes de este miembro	Aug 23 2008, 03:35 PM Publicado: #2542
Maestro Matemático Grupo: Usuario FMAT Mensajes: 115 Registrado: 20-May 08 Miembro Nº: 23.908 Nacionalidad: Colegio/Liceo: Universidad: Sexo:	$TEX: $\begin{gathered}<br /> P.10 \hfill \\<br /> \hfill \\<br /> f(x) = \sqrt {(x - 3)^2 } \xrightarrow{{}}f(x) = \left\| {x - 3} \right\| \hfill \\<br /> \hfill \\<br /> La{\text{ funcion valor Absoluto esta dada por:}} \hfill \\<br /> \hfill \\<br /> f(x) = {\text{ }}\left\| x \right\| + a{\text{ }}{\text{, }}a{\text{ }}\rangle {\text{ 0 - }}f(x) = {\text{ }}\left\| x \right\| + a{\text{ }}{\text{, }}a{\text{ }}\langle {\text{ 0}} \hfill \\<br /> {\text{Donde Indica el punto de traslacion en el eje de las ordenadas}} \hfill \\<br /> \hfill \\<br /> f(x) = \left\| {x - b} \right\|{\text{ }}{\text{, b }}\langle {\text{ 0 - }}f(x) = \left\| {x - b} \right\|{\text{ }}{\text{, b }}\rangle {\text{ 0 }} \hfill \\<br /> {\text{Donde Indica el punto de traslacion en el eje de las abscisas}}{\text{.}} \hfill \\<br /> \hfill \\<br /> {\text{Conociendo esto podemos concluir que la grafica de valor absoluto de la funcion :}} \hfill \\<br /> f(x) = \left\| {x - 3} \right\|,{\text{ esta Dada en la alternativa A}} \hfill \\<br /> \hfill \\<br /> Alternativa{\text{ A}}{\text{.}} \hfill \\ <br />\end{gathered} $<br />$ -------------------- Estudiante de Segundo Año Universidad Técnica Federico Santa María

angelita Ver Perfil del Miembro Ver los mensajes de este miembro	Aug 23 2008, 04:52 PM Publicado: #2543
Dios Matemático Grupo: Usuario FMAT Mensajes: 363 Registrado: 12-October 06 Miembro Nº: 2.478 Nacionalidad: Colegio/Liceo: Universidad: Sexo:	$TEX: \[<br />\begin{gathered}<br /> {\text{16}}{\text{. Cual (es) de las siguientes afirmaciones es (son) verdadera (s) con respecto}} \hfill \\<br /> {\text{a la recta y - x - 2 = 0?}} \hfill \\<br /> {\text{I}}{\text{. La recta intersecta al eje y en el punto (0}}{\text{,2)}} \hfill \\<br /> {\text{Verdadero}} \hfill \\<br /> \boxed\begin{gathered}<br /> {\text{La recta es }}y = x + 2 \hfill \\<br /> y = 0 + 2 \hfill \\<br /> y = 2 \hfill \\<br /> x = 0 \hfill \\ <br />\end{gathered} \hfill \\<br /> \hfill \\<br /> {\text{II}}{\text{. La recta intesecta al eje x en el punto ( - 2}}{\text{,0)}} \hfill \\<br /> {\text{Verdadero}} \hfill \\<br /> \boxed\begin{gathered}<br /> y = x + 2 \hfill \\<br /> y = - 2 + 2 \hfill \\<br /> y = 0 \hfill \\<br /> x = - 2 \hfill \\ <br />\end{gathered} \hfill \\<br /> III.{\text{ La pendiente de la recta es positiva}} \hfill \\<br /> {\text{verdadero}} \hfill \\<br /> \boxed\begin{gathered}<br /> {\text{y = }}mx + b \hfill \\<br /> m = pendiente \hfill \\<br /> y = x + 2 \hfill \\<br /> m = 1 \hfill \\ <br />\end{gathered} \hfill \\<br /> \hfill \\<br /> \boxed{{\text{Alternativa E}}} \hfill \\ <br />\end{gathered} <br />\]$

angelita Ver Perfil del Miembro Ver los mensajes de este miembro	Aug 23 2008, 04:56 PM Publicado: #2544
Dios Matemático Grupo: Usuario FMAT Mensajes: 363 Registrado: 12-October 06 Miembro Nº: 2.478 Nacionalidad: Colegio/Liceo: Universidad: Sexo:	$TEX: \[<br />\begin{gathered}<br /> {\text{16}}{\text{. Cual (es) de las siguientes afirmaciones es (son) verdadera (s) con respecto}} \hfill \\<br /> {\text{a la recta y - x - 2 = 0?}} \hfill \\<br /> {\text{I}}{\text{. La recta intersecta al eje y en el punto (0}}{\text{,2)}} \hfill \\<br /> {\text{Verdadero}} \hfill \\<br /> {\text{La recta es }}y = x + 2 \hfill \\<br /> y = 0 + 2 \hfill \\<br /> y = 2 \hfill \\<br /> x = 0 \hfill \\<br /> {\text{II}}{\text{. La recta intesecta al eje x en el punto ( - 2}}{\text{,0)}} \hfill \\<br /> {\text{Verdadero}} \hfill \\<br /> y = x + 2 \hfill \\<br /> y = - 2 + 2 \hfill \\<br /> y = 0 \hfill \\<br /> x = - 2 \hfill \\<br /> III.{\text{ La pendiente de la recta es positiva}} \hfill \\<br /> {\text{verdadero}} \hfill \\<br /> {\text{y = }}mx + b \hfill \\<br /> m = pendiente \hfill \\<br /> y = x + 2 \hfill \\<br /> m = 1 \hfill \\<br /> \boxed{{\text{Alternativa E}}} \hfill \\ <br />\end{gathered} <br />\]$

Brodo Ver Perfil del Miembro Ver los mensajes de este miembro	Aug 23 2008, 07:17 PM Publicado: #2545
Dios Matemático Grupo: Usuario FMAT Mensajes: 297 Registrado: 27-May 07 Desde: Strawberry Fields Miembro Nº: 6.208 Nacionalidad: Colegio/Liceo: Universidad: Sexo:	$TEX: \[<br />\begin{gathered}<br /> 12.{\text{ }}\int {\frac{{dx}}<br />{{\sqrt {x^2 - 2} }}} \hfill \\<br /> 13.{\text{ }}\int {\left( {x^2 - x} \right)} e^{3x} {\text{ }}dx \hfill \\<br /> 14.{\text{ }}\int {\frac{{dx}}<br />{{(4x^2 + 4x^3 + x^4 )}}} \hfill \\<br /> 15.{\text{ }}\int {\log \left( {3x + x^2 } \right)} {\text{ }}dx \hfill \\ <br />\end{gathered} <br />\]<br />$ -------------------- ada Para Declarar......

Todo sea por la ... Todo sea por la causa Ver Perfil del Miembro Ver los mensajes de este miembro	Aug 23 2008, 07:32 PM Publicado: #2546
Dios Matemático Grupo: Usuario FMAT Mensajes: 270 Registrado: 7-August 07 Miembro Nº: 8.312 Nacionalidad: Colegio/Liceo: Universidad: Sexo:	raiz de (x-2) Mensaje modificado por Todo sea por la causa el Aug 23 2008, 08:03 PM -------------------- Llegó el momento de la ansiada despedida :=)

Manuel Fco Ver Perfil del Miembro Ver los mensajes de este miembro	Aug 23 2008, 08:21 PM Publicado: #2547
Dios Matemático Supremo Grupo: Usuario FMAT Mensajes: 1.077 Registrado: 19-December 07 Desde: Santiago, RM. Miembro Nº: 13.971 Nacionalidad: Colegio/Liceo: Universidad: Sexo:	$TEX: \[<br />\begin{gathered}<br /> m_1 v_1 + m_2 v_2 = m_1 v_1 ' + m_2 v_2 ' \hfill \\<br /> m_2 v_2 - m_2 v_2 ' = m_1 v_1 ' - m_1 v_1 \hfill \\<br /> m_2 (v_2 - v_2 ') = m_1 v_1 ' - m_1 v_1 \hfill \\<br /> m_2 = \frac{{m_1 v_1 ' - m_1 v_1 }}<br />{{(v_2 - v_2 ')}} \hfill \\ <br />\end{gathered} <br />\]<br />$

prog__guitar Ver Perfil del Miembro Ver los mensajes de este miembro	Aug 23 2008, 08:41 PM Publicado: #2548
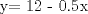
Doctor en Matemáticas Grupo: Usuario FMAT Mensajes: 161 Registrado: 29-December 07 Miembro Nº: 14.172 Nacionalidad: Colegio/Liceo: Universidad: Sexo:	$TEX: y= 12 - 0.5x$ Mensaje modificado por speed__guitar el Aug 23 2008, 08:42 PM

Tanteador Ver Perfil del Miembro Ver los mensajes de este miembro	Aug 23 2008, 11:39 PM Publicado: #2549
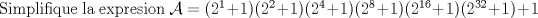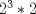
Principiante Matemático Destacado Grupo: Usuario FMAT Mensajes: 20 Registrado: 9-May 06 Miembro Nº: 1.055	$TEX: \noindent Simplifique la expresion $\mathcal{A}=(2^1+1)(2^2+1)(2^4+1)(2^8+1)(2^{16}+1)(2^{32}+1)+1$$ $TEX: $2^3*2$$

pingu Ver Perfil del Miembro Ver los mensajes de este miembro	Aug 24 2008, 12:27 PM Publicado: #2550
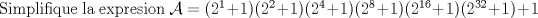
Principiante Matemático Destacado Grupo: Usuario FMAT Mensajes: 26 Registrado: 22-August 08 Miembro Nº: 32.840 Nacionalidad: Universidad: Sexo:	$TEX: \noindent Simplifique la expresion $\mathcal{A}=(2^1+1)(2^2+1)(2^4+1)(2^8+1)(2^{16}+1)(2^{32}+1)+1$$

« Posterior · Sector Manuales · Anterior »

437 Páginas:

« < 253 254 255 256 257 > »

2 usuario(s) está(n) leyendo esta discusión (2 invitado(s) y 0 usuario(s) anónimo(s))

0 miembro(s):

Modo de Ver: Estándar · Cambiar a: Lineal+ · Cambiar a: Outline

Suscribirse · Enviar por correo · Imprimir · Suscribirse a este foro

Versión Lo-Fi

Fecha y Hora actual: 27th November 2024 - 05:45 AM