Probando LaTeX - Foro fmat.cl

Portal Matemático

¿Qué es FMAT?

Foro fmat.cl > Sector Comunidad Fmat > Sector Manuales

437 Páginas:

« < 14 15 16 17 18 > »

Closed Topic

Start new topic

Probando LaTeX

gotcha Ver Perfil del Miembro Ver los mensajes de este miembro	May 31 2006, 10:09 PM Publicado: #151
Principiante Matemático Grupo: Usuario FMAT Mensajes: 7 Registrado: 7-August 05 Desde: Frente al pc Miembro Nº: 177 Nacionalidad: Sexo:	$TEX: Sea $f:$ $X \to Y$. Sean $A, B \subseteq X$. Demuestre que: \newline<br />a) $f$ ($\bigcup_k$ $A_k$) = $\bigcup_k f$($A_k$) \newline<br />b) $f$ ($\bigcap_k A_k$) $\subseteq$ $\bigcap_k f$($A_k$)$ probando una pregunta de una guía -------------------- Blog: http://gotcha.distro.cl

Zirou Ver Perfil del Miembro Ver los mensajes de este miembro	Jun 2 2006, 06:29 PM Publicado: #152
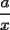
Máquina que convierte café en teoremas Grupo: Colaborador Silver Mensajes: 1.665 Registrado: 18-August 05 Desde: Concepción Miembro Nº: 247 Nacionalidad: Colegio/Liceo: Universidad: Sexo:	$TEX: $\displaystyle \frac{a}{x}$$ -------------------- $TEX: $mathcal{Z}$ $imath$ $Re$ $varnothing$ $mho$$ Manual para subir imágenes y archivos a fmat (con servidor propio) Manual de latex Estilo Propio Lista de libros en fmat "Un Matemático es una máquina que trasforma café en teoremas"(Erdös) --- Consultas, sugerencias, reclamos via mp o a los correos mencionados.

gotcha Ver Perfil del Miembro Ver los mensajes de este miembro	Jun 5 2006, 01:23 PM Publicado: #153
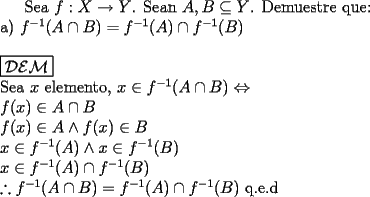Principiante Matemático Grupo: Usuario FMAT Mensajes: 7 Registrado: 7-August 05 Desde: Frente al pc Miembro Nº: 177 Nacionalidad: Sexo:	$TEX: Sea $f: X \rightarrow Y$. Sean $A, B \subseteq Y$. Demuestre que: \newline<br />a) $f^{-1}(A \cap B) = f^{-1}(A) \cap f^{-1}(B)$ \newline \newline<br />$\boxed{\mathcal{DEM}}$ \newline <br />Sea $x$ elemento,<br />$x \in f^{-1}(A \cap B) \Leftrightarrow $ \newline<br />$ f(x) \in A \cap B$ \newline<br />$ f(x) \in A \wedge f(x) \in B$ \newline<br />$x \in f^{-1}(A) \wedge x \in f^{-1}(B)$ \newline<br />$x \in f^{-1}(A) \cap f^{-1}(B)$ \newline<br />$\therefore f^{-1}(A \cap B) = f^{-1}(A) \cap f^{-1}(B)$<br />q.e.d <br /><br />$ ] una tarea -------------------- Blog: http://gotcha.distro.cl

Killua Ver Perfil del Miembro Ver los mensajes de este miembro	Jun 6 2006, 02:07 PM Publicado: #154
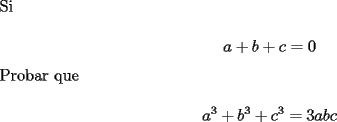Staff Fmat Grupo: Moderador Mensajes: 1.185 Registrado: 29-October 05 Desde: Santiago, Chile Miembro Nº: 352 Nacionalidad: Colegio/Liceo: Universidad: Sexo:	$TEX: \noindent Si<br /><br />$$a+b+c=0$$<br /><br />\noindent Probar que<br /><br />$$a^3+b^3+c^3=3abc$$$ $TEX: $Excelente!!!!!$$ Saludos -------------------- "He looks rather ill, but he looks all over the genius he was" (G. H. Hardy) "A mathematician is a device for turning coffee into theorems" (Paul Erdös)

Santis Ver Perfil del Miembro Ver los mensajes de este miembro	Jun 6 2006, 08:09 PM Publicado: #155
Maestro Matemático Grupo: Usuario FMAT Mensajes: 80 Registrado: 6-June 06 Desde: Argentina Miembro Nº: 1.263 Nacionalidad: Sexo:	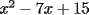$TEX: $x^2-7x+15$$ Cuadrado de Binomio 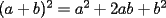$TEX: $(a+b)^2=a^2+2ab+b^2$$ --------------------

Kwisatz Haderach Ver Perfil del Miembro Ver los mensajes de este miembro	Jun 9 2006, 09:21 PM Publicado: #156
Principiante Matemático Grupo: Usuario FMAT Mensajes: 4 Registrado: 17-May 06 Miembro Nº: 1.110	[text]Sea $f: \mathbb{R} \rightarrow \mathbb{R}$ que verifica $f(n+m)=f(n) \cdot f(m)$. Si $f(2)=2$, calcule $f(2005)$. Justifique[/text]

Kwisatz Haderach Ver Perfil del Miembro Ver los mensajes de este miembro	Jun 9 2006, 09:24 PM Publicado: #157
Principiante Matemático Grupo: Usuario FMAT Mensajes: 4 Registrado: 17-May 06 Miembro Nº: 1.110	$TEX: Sea $f: \mathbb{R} \rightarrow \mathbb{R}$ que verifica $f(n+m)=f(n) \cdot f(m)$. Si $f(2)=2$, calcule $f(2005)$. Justifique$

Kwisatz Haderach Ver Perfil del Miembro Ver los mensajes de este miembro	Jun 9 2006, 09:29 PM Publicado: #158
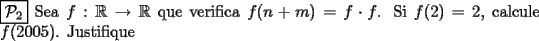
Principiante Matemático Grupo: Usuario FMAT Mensajes: 4 Registrado: 17-May 06 Miembro Nº: 1.110	$TEX: \noindent $\boxed{\mathcal{P}_{2}}$ Sea $f: \mathbb{R} \rightarrow \mathbb{R}$ que verifica $f(n+m)=f \cdot f $. Si $f(2)=2$, calcule $f(2005)$. Justifique$

Kwisatz Haderach Ver Perfil del Miembro Ver los mensajes de este miembro	Jun 9 2006, 11:09 PM Publicado: #159
Principiante Matemático Grupo: Usuario FMAT Mensajes: 4 Registrado: 17-May 06 Miembro Nº: 1.110	$TEX: $\boxed{\mathcal{P}_{2}}$ Respuesta: $f(1+1)=2$ luego $f(1)^2=2$ (propiedad) luego $f(1)=2^\frac{1}{2}$ luego $f(2005)=f(2004) \cdot f(1)$ entonces $f(2005)=f(2)^{1002} \cdot f(1)$ reemplazando $f(2005)=2^{1002} \cdot 2^\frac{1}{2}$ y $f(2005)=2^\frac{2005}{2}$$ $TEX: Probemos que: $f(x)=2^\frac{x}{2}$ demostracion(por induccion): $x=1$ $f(1+1)=2$ desarrollando(propiedad): $f(1)=2^\frac{1}{2}$ , lo cual respalda a $f(x)=2^\frac{x}{2}$ para $x=1$ Supongamos verdadero $f(n)=2^\frac{n}{2}$ , demostremos que se cumple para $n+1$ $f(n+1)=f(n) \cdot f(1)$ pero como $f(n)=2^\frac{n}{2}$ y $f(1)=2^\frac{1}{2}$ tenemos que: $f(n)=2^\frac{n}{2} \cdot 2^\frac{1}{2}$ entonces $f(n+1)=2^\frac{n+1}{2}$ lo cual comprueba la formula: $f(x)=2^\frac{x}{2}$ , ahora aplicando la formula: $f(2005)=2^\frac{2005}{2}$$ esto es una respuesta pa un foro q no tiene latex, por eso la posteo aca pa despùes poder pegarla

gotcha Ver Perfil del Miembro Ver los mensajes de este miembro	Jun 9 2006, 11:15 PM Publicado: #160
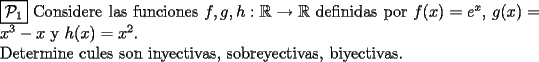
Principiante Matemático Grupo: Usuario FMAT Mensajes: 7 Registrado: 7-August 05 Desde: Frente al pc Miembro Nº: 177 Nacionalidad: Sexo:	$TEX: \noindent $\boxed{\mathcal{P}_{1}}$ Considere las funciones $f, g, h : \mathbb{R} \rightarrow \mathbb{R}$ definidas por $f(x)=e^x$, $g(x)=x^3 - x$ y $h(x)=x^2$. \newline Determine cuáles son inyectivas, sobreyectivas, biyectivas.$ $TEX: \noindent $\boxed{\mathcal{S}_{P1}}$ Vemos caso a caso. \newline <br />a) Comencemos con: $f: \mathbb{R} \rightarrow \mathbb{R}$ tal que, $x \mapsto f(x) =e^x$. \newline Tenemos que el $Dom(f)$=$\mathbb{R}$ , y su $Rec(f)$=$\mathbb{R}$. \newline ¿ Es $f$ inyectiva (1-1)? Si $f$ es inyectiva, entonces, \newline $( \forall x, y \in \mathbb{R}) f(x) = f(y) \Leftrightarrow x = y$ \newline es decir, para cualquier par de elementos $x, y$ que pertenecen al dominio($\mathbb{R}$), si la funci$\acute{o}$n $f$ evaluado en cada uno de ellos est$\acute{a}$ asociado al mismo elemento, entonces, x e y, son el mismo elemento. \newline Más formalmente podemos demostrar que $f$ es inyectiva: <br />\newline Sean $x,y \in \mathbb{R}$ y $f$ funci$\acute{o}$n anteriormente definida, tal que $f$ es inyectiva(supuesto).\newline<br />$f(x)=f(y)$ \newline<br />$e^x=e^y \diagup ln()$ \newline<br />$ln(e^x)=ln(e^y)$ \newline<br />$x$$\cdot$$ln(e)$=$y$$\cdot$$ln(e)$ \newline<br />$x=y$ \newline<br />Por tanto, $f$ es inyectiva.\newline<br />Ahora probemos si $f$ es sobreyectiva. Si $f$ es sobreyectiva, entonces se cumple que $Im(f)$=$Rec(f)$, evaluando para este caso en particular, tenemos que: $(0,\infty) \neq \mathbb{R}$. Por lo tanto, $f$ no es sobreyectiva. \newline<br />Si $f$ es inyectiva, pero no es sobreyectiva, no es biyectiva.\newline \newline<br />b) Se tiene $g: \mathbb{R} \rightarrow \mathbb{R}$, $x \mapsto$ $g(x)=x^3 - x$. \newline Para este desarrollo basemonos en la siguente gr$\acute{a}$fica:$ $TEX: Claramente vemos que si trazamos una recta paralela al eje $X$, esta corta la funci$\acute{o}$n en 1, 2 o 3 puntos. Esto significa que para una imagen $y$ existe m$\acute{a}$s de una preimagen asociada, por tanto $g$ no es inyectiva. \newline<br />Ahora, para que $g$ sea sobreyectiva, se debe cumplir que: \newline<br />$Im(g)$=$Rec(g)$ \newline<br />en otras palabras, \newline<br />$(\forall b \in Rec(g))(\exists a \in Dom(g)) b = f(a)$ \newline<br />Es decir: Para cualquier elemento $b$ dentro del recorrido de $g$, siempre hay al menos un elemento $a$ que es su preimagen. <br />Y claramente podemos decir que $g$ es sobreyectiva al mirar la gráfica, ya que el eje $Y$(recorrido) tiene asociado siempre un elemento en el eje $X$ (dominio). \newline Ahora, como $g$ es sobreyectiva pero no inyectiva, $g$ no es biyectiva.<br />\newline \newline<br />c) Sea $h: \mathbb{R} \rightarrow \mathbb{R}$ , $ x \mapsto$ $h(x)=x^2$. \newline Inyectividad: $h$ no es inyectiva ya que $f(+x)=f(-x)=x^2$ y claramente $+x \neq -x$, asi tenemos dos elementos distintos con la misma imagen asociada.\newline Sobreyectividad: $h$ no es sobreyectiva ya que $Im(h) \neq Rec(h)$ donde $\mathbb{R}^{+} \neq \mathbb{R}$, por lo tanto $h$ no es sobreyectiva.\newline Al no ser inyectiva ni sobreyectiva, no es biyectiva.$ -------------------- Blog: http://gotcha.distro.cl

« Posterior · Sector Manuales · Anterior »

437 Páginas:

« < 14 15 16 17 18 > »

Closed Topic

Start new topic

1 usuario(s) está(n) leyendo esta discusión (1 invitado(s) y 0 usuario(s) anónimo(s))

0 miembro(s):

Modo de Ver: Estándar · Cambiar a: Lineal+ · Cambiar a: Outline

Suscribirse · Enviar por correo · Imprimir · Suscribirse a este foro

Versión Lo-Fi

Fecha y Hora actual: 30th October 2025 - 12:34 PM

Powered By IP.Board 2.2.1 © 2007 IPS, Inc.